| CONDENSED MATTER: ELECTRONIC STRUCTURE, ELECTRICAL, MAGNETIC, AND OPTICAL PROPERTIES |
 |
|

|
|
|
Consistent Scaling Exponents at the Deconfined Quantum-Critical Point |
| Anders W. Sandvik1,2**, Bowen Zhao1 |
1Department of Physics, Boston University, Boston, Massachusetts 02215, USA
2Beijing National Laboratory for Condensed Matter Physics and Institute of Physics, Chinese Academy of Sciences, Beijing 100190
|
|
| Cite this article: |
|
Anders W. Sandvik, Bowen Zhao 2020 Chin. Phys. Lett. 37 057502 |
|
|
|
|
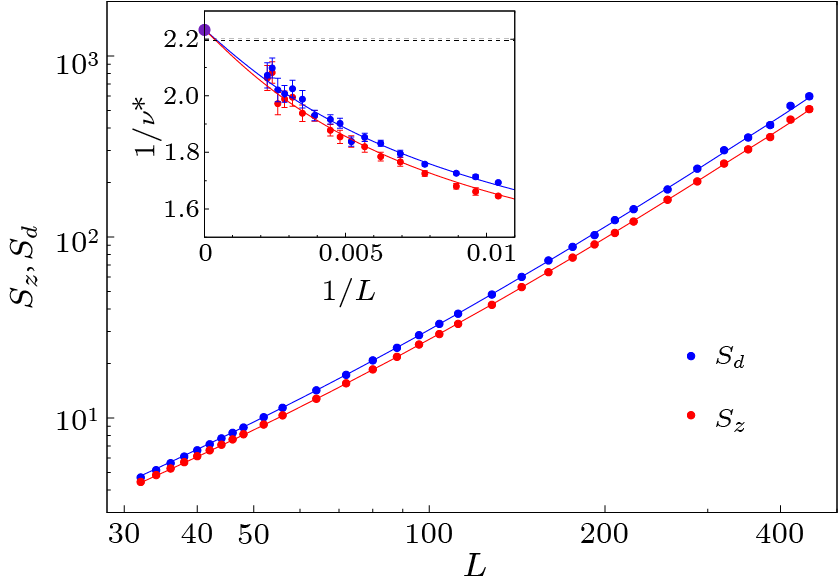
Abstract We report a quantum Monte Carlo study of the phase transition between antiferromagnetic and valence-bond solid ground states in the square-lattice $S=1/2$ $J$–$Q$ model. The critical correlation function of the $Q$ terms gives a scaling dimension corresponding to the value $\nu = 0.455 \pm 0.002$ of the correlation-length exponent. This value agrees with previous (less precise) results from conventional methods, e.g., finite-size scaling of the near-critical order parameters. We also study the $Q$-derivatives of the Binder cumulants of the order parameters for $L^2$ lattices with $L$ up to $448$. The slope grows as $L^{1/\nu}$ with a value of $\nu$ consistent with the scaling dimension of the $Q$ term. There are no indications of runaway flow to a first-order phase transition. The mutually consistent estimates of $\nu$ provide compelling support for a continuous deconfined quantum-critical point.
|
|
Received: 06 April 2020
Published: 21 April 2020
|
|
| PACS: |
75.10.Jm
|
(Quantized spin models, including quantum spin frustration)
|
| |
64.70.Tg
|
(Quantum phase transitions)
|
| |
75.40.Mg
|
(Numerical simulation studies)
|
| |
75.30.Kz
|
(Magnetic phase boundaries (including classical and quantum magnetic transitions, metamagnetism, etc.))
|
|
|
| Fund: Supported by the National Science Foundation (USA) under Grant No. DMR-1710170 and by the Simons Foundation under a Simons Investigator Award. |
|
|
|
| [1] | Sachdev S 2008 Nat. Phys. 4 173 | | [2] | Savary L and Balents L 2017 Rep. Prog. Phys. 80 016502 | | [3] | Wen X G 2019 Science 363 eaal3099 | | [4] | Senthil T, Vishwanath A, Balents L, Sachdev S and Fisher M P A 2004 Science 303 1490 | | [5] | Kaul R K, Melko R G and Sandvik A W 2013 Annu. Rev. Condens. Matter Phys. 4 179 | | [6] | Sandvik A W, Daul S, Singh R R P and Scalapino D J 2002 Phys. Rev. Lett. 89 247201 | | [7] | Haldane F D M 1988 Phys. Rev. Lett. 61 1029 | | [8] | Chakravarty S, Halperin B I and Nelson D R 1989 Phys. Rev. B 39 2344 | | [9] | Read N and Sachdev S 1989 Phys. Rev. Lett. 62 1694 | | [10] | Read N and Sachdev S 1990 Phys. Rev. B 42 4568 | | [11] | Dagotto E and Moreo A 1989 Phys. Rev. Lett. 63 2148 | | [12] | Murthy G and Sachdev S 1990 Nucl. Phys. B 344 557 | | [13] | Motrunich O I and Vishwanath A 2004 Phys. Rev. B 70 075104 | | [14] | Sandvik A W 2007 Phys. Rev. Lett. 98 227202 | | [15] | Melko R G and Kaul R K 2008 Phys. Rev. Lett. 100 017203 | | [16] | Jiang F J, Nyfeler M, Chandrasekharan S and Wiese U J 2008 J. Stat. Mech.: Theory Exp. 2008 P02009 | | [17] | Lou J, Sandvik A W and Kawashima N 2009 Phys. Rev. B 80 180414 | | [18] | Sandvik A W 2010 Phys. Rev. Lett. 104 177201 | | [19] | Kaul R K 2011 Phys. Rev. B 84 054407 | | [20] | Sandvik A W 2012 Phys. Rev. B 85 134407 | | [21] | Harada K, Suzuki T, Okubo T, Matsuo H, Lou J, Watanabe H, Todo S and Kawashima N 2013 Phys. Rev. B 88 220408 | | [22] | Chen K, Huang Y, Deng Y, Kuklov A B, Prokof'ev N V and Svistunov B V 2013 Phys. Rev. Lett. 110 185701 | | [23] | Block M S, Melko R G and Kaul R K 2013 Phys. Rev. Lett. 111 137202 | | [24] | Pujari S, Alet F and Damle K 2015 Phys. Rev. B 91 104411 | | [25] | Suwa H, Sen A and Sandvik A W 2016 Phys. Rev. B 94 144416 | | [26] | Shao H, Guo W and Sandvik A W 2016 Science 352 213 | | [27] | Ma N, Sun G Y, You Y Z, Xu C, Vishwanath A, Sandvik A W and Meng Z Y 2018 Phys. Rev. B 98 174421 | | [28] | Evertz H G 2003 Adv. Phys. 52 1 | | [29] | Sandvik A W 1999 Phys. Rev. B 59 R14157 | | [30] | Sandvik A W 2010 AIP Conf. Proc. 1297 135 | | [31] | Nahum A, Serna P, Chalker J T, Ortuño M and Somoza A M 2015 Phys. Rev. Lett. 115 267203 | | [32] | Nahum A, Chalker J T, Serna P, Ortuño M and Somoza A M 2015 Phys. Rev. X 5 041048 | | [33] | Zhao B, Weinberg P and Sandvik A W 2019 Nat. Phys. 15 678 | | [34] | Serna P and Nahum A 2019 Phys. Rev. B 99 195110 | | [35] | Takahashi J and Sandvik A W 2020 arXiv:2001.10045 | | [36] | Kuklov A B, Matsumoto M, Prokof’ev N V, Svistunov B V, Troyer M 2008 Phys. Rev. Lett. 101 050405 | | [37] | Levin M and Senthil T 2004 Phys. Rev. B 70 220403 | | [38] | Wang C, Nahum A, Metlitski M A, Xu C and Senthil T 2017 Phys. Rev. X 7 031051 | | [39] | Ma H and He Y C 2019 Phys. Rev. B 99 195130 | | [40] | Ma R and Wang C 2019 arXiv:1912.12315 | | [41] | Nahum A 2019 arXiv:1912.13468 | | [42] | Gorbenko V, Rychkov S and Zan B 2018 J. High Energy Phys. 2018(10) 108 | | [43] | Gorbenko V, Rychkov S and Zan B 2018 SciPost Phys. 5 050 | | [44] | Patil P, Tang Y, Katz E and Sandvik A W 2017 Phys. Rev. B 96 045140 | | [45] | Sandvik A W and Kurkijärvi J 1991 Phys. Rev. B 43 5950 | | [46] | Sandvik A W 1992 J. Phys. A: Math. Gen. 25 3667 | | [47] | Wang L, Beach K S D and Sandvik A W 2006 Phys. Rev. B 73 014431 | | [48] | Sen A, Suwa H and Sandvik A W 2015 Phys. Rev. B 92 195145 | | [49] | Campostrini M, Hasenbusch M, Pelissetto A, Rossi P and Vicari E 2002 Phys. Rev. B 65 144520 | | [50] | Jiang F J and Wiese U J 2011 Phys. Rev. B 83 155120 | | [51] | Iino S, Morita S, Kawashima N and Sandvik A W 2019 J. Phys. Soc. Jpn. 88 034006 | | [52] | Nakayama Y and Ohtsuki T 2016 Phys. Rev. Lett. 117 131601 | | [53] | Liu Y H, Wang Z J, Sato T, Hohenadler M, Wang C, Guo W A and Assaad F F 2019 Nat. Commun. 10 2658 |
|
|
Viewed |
|
|
|
Full text
|
|
|
|
|
Abstract
|
|
|
|
|