| CONDENSED MATTER: ELECTRONIC STRUCTURE, ELECTRICAL, MAGNETIC, AND OPTICAL PROPERTIES |
 |
|

|
|
|
Learning the Effective Spin Hamiltonian of a Quantum Magnet |
| Sizhuo Yu1†, Yuan Gao1†, Bin-Bin Chen1, and Wei Li2,1* |
1School of Physics, Beihang University, Beijing 100191, China
2CAS Key Laboratory of Theoretical Physics, Institute of Theoretical Physics, Chinese Academy of Sciences, Beijing 100190, China
|
|
| Cite this article: |
|
Sizhuo Yu, Yuan Gao, Bin-Bin Chen et al 2021 Chin. Phys. Lett. 38 097502 |
|
|
|
|
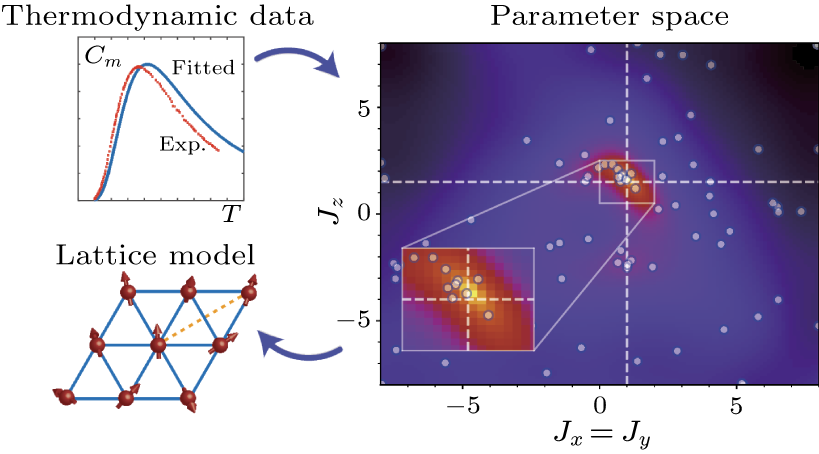
Abstract To understand the intriguing many-body states and effects in the correlated quantum materials, inference of the microscopic effective Hamiltonian from experiments constitutes an important yet very challenging inverse problem. Here we propose an unbiased and efficient approach learning the effective Hamiltonian through the many-body analysis of the measured thermal data. Our approach combines the strategies including the automatic gradient and Bayesian optimization with the thermodynamics many-body solvers including the exact diagonalization and the tensor renormalization group methods. We showcase the accuracy and powerfulness of the Hamiltonian learning by applying it firstly to the thermal data generated from a given spin model, and then to realistic experimental data measured in the spin-chain compound copper nitrate and triangular-lattice magnet TmMgGaO$_4$. The present automatic approach constitutes a unified framework of many-body thermal data analysis in the studies of quantum magnets and strongly correlated materials in general.
|
|
Received: 10 August 2021
Express Letter
Published: 27 August 2021
|
|
| PACS: |
75.10.Dg
|
(Crystal-field theory and spin Hamiltonians)
|
| |
75.10.Jm
|
(Quantized spin models, including quantum spin frustration)
|
| |
75.40.Mg
|
(Numerical simulation studies)
|
| |
05.70.-a
|
(Thermodynamics)
|
|
|
| Fund: Supported by the National Natural Science Foundation of China (Grant Nos. 11974036 and 11834014). |
|
|
|
| [1] | Anderson P W 1973 Mater. Res. Bull. 8 153 |
| [2] | Kitaev A 2006 Ann. Phys. 321 2 |
| [3] | Zhou Y, Kanoda K, and Ng T K 2017 Rev. Mod. Phys. 89 025003 |
| [4] | Balents L 2010 Nature 464 199 |
| [5] | Han T H, Helton J S, Chu S, Nocera D G, Rodriguez-Rivera J A, Broholm C, and Lee Y S 2012 Nature 492 406 |
| [6] | Fu M, Imai T, Han T H, and Lee Y S 2015 Science 350 655 |
| [7] | Feng Z, Yi W, Zhu K, Wei Y, Miao S, Ma J, Luo J, Li S, Meng Z Y, and Shi Y 2019 Chin. Phys. Lett. 36 017502 |
| [8] | Shimizu Y, Miyagawa K, Kanoda K, Maesato M, and Saito G 2003 Phys. Rev. Lett. 91 107001 |
| [9] | Yamashita M, Nakata N, Senshu Y, Nagata M, Yamamoto H M, Kato R, Shibauchi T, and Matsuda Y 2010 Science 328 1246 |
| [10] | Liu W, Zhang Z, Ji J, Liu Y, Li J, Wang X, Lei H, Chen G, and Zhang Q 2018 Chin. Phys. Lett. 35 117501 |
| [11] | Jia Y T, Gong C S, Liu Y X, Zhao J F, Dong C, Dai G Y, Li X D, Lei H C, Yu R Z, Zhang G M, and Jin C Q 2020 Chin. Phys. Lett. 37 097404 |
| [12] | Zhang Z, Li J, Liu W, Zhang Z, Ji J, Jin F, Chen R, Wang J, Wang X, Ma J, and Zhang Q 2021 Phys. Rev. B 103 184419 |
| [13] | Jackeli G and Khaliullin G 2009 Phys. Rev. Lett. 102 017205 |
| [14] | Chaloupka J, Jackeli G, and Khaliullin G 2010 Phys. Rev. Lett. 105 027204 |
| [15] | Ye F, Chi S, Cao H, Chakoumakos B C, Fernandez-Baca J A, Custelcean R, Qi T F, Korneta O B, and Cao G 2012 Phys. Rev. B 85 180403(R) |
| [16] | Banerjee A, Bridges C A, Yan J Q, Aczel A A, Li L, Stone M B, Granroth G E, Lumsden M D, Yiu Y, Knolle J, Bhattacharjee S, Kovrizhin D L, Moessner R, Tennant D A, Mandrus D G, and Nagler S E 2016 Nat. Mater. 15 733 |
| [17] | Banerjee A, Yan J, Knolle J, Bridges C A, Stone M B, Lumsden M D, Mandrus D G, Tennant D A, Moessner R, and Nagler S E 2017 Science 356 1055 |
| [18] | Sears J A, Chern L E, Kim S, Bereciartua P J, Francoual S, Kim Y B, and Kim Y J 2020 Nat. Phys. 16 837 |
| [19] | Banerjee A, Lampen-Kelley P, Knolle J, Balz C, Aczel A, Winn B, Liu Y, Pajerowski D, Yan J, Bridges C A, Savici A T, Chakoumakos B C, Lumsden M D, Tennant D A, Moessner R, Mandrus D G, and Nagler S E 2018 npj Quantum Mater. 3 8 |
| [20] | Kubota Y, Tanaka H, Ono T, Narumi Y, and Kindo K 2015 Phys. Rev. B 91 094422 |
| [21] | Do S H, Park S Y, Yoshitake J, Nasu J, Motome Y, Kwon Y S, Adroja D T, Voneshen D J, Kim K, Jang T H, Park J H, Choi K Y, and Ji S 2017 Nat. Phys. 13 1079 |
| [22] | Widmann S, Tsurkan V, Prishchenko D A, Mazurenko V G, Tsirlin A A, and Loidl A 2019 Phys. Rev. B 99 094415 |
| [23] | Lampen-Kelley P, Rachel S, Reuther J, Yan J Q, Banerjee A, Bridges C A, Cao H B, Nagler S E, and Mandrus D 2018 Phys. Rev. B 98 100403 |
| [24] | Weber D, Schoop L M, Duppel V, Lippmann J M, Nuss J, and Lotsch B V 2016 Nano Lett. 16 3578 |
| [25] | Johnson R D, Williams S C, Haghighirad A A, Singleton J, Zapf V, Manuel P, Mazin I I, Li Y, Jeschke H O, Valentí R, and Coldea R 2015 Phys. Rev. B 92 235119 |
| [26] | Laurell P and Okamoto S 2020 npj Quantum Mater. 5 2 |
| [27] | Wiebe N, Granade C, Ferrie C, and Cory D G 2014 Phys. Rev. Lett. 112 190501 |
| [28] | Sels D, Dashti H, Mora S, Demler O, and Demler E 2020 Nat. Mach. Intell. 2 396 |
| [29] | Pakrouski K 2020 Quantum 4 315 |
| [30] | Bursill R J, Xiang T, and Gehring G A 1996 J. Phys.: Condens. Matter 8 L583 |
| [31] | Wang X and Xiang T 1997 Phys. Rev. B 56 5061 |
| [32] | Xiang T 1998 Phys. Rev. B 58 9142 |
| [33] | Feiguin A E and White S R 2005 Phys. Rev. B 72 220401(R) |
| [34] | White S R 2009 Phys. Rev. Lett. 102 190601 |
| [35] | Stoudenmire E M and White S R 2010 New J. Phys. 12 055026 |
| [36] | Li W, Ran S J, Gong S S, Zhao Y, Xi B, Ye F, and Su G 2011 Phys. Rev. Lett. 106 127202 |
| [37] | Dong Y L, Chen L, Liu Y J, and Li W 2017 Phys. Rev. B 95 144428 |
| [38] | Chen B B, Liu Y J, Chen Z, and Li W 2017 Phys. Rev. B 95 161104(R) |
| [39] | Chen B B, Chen L, Chen Z, Li W, and Weichselbaum A 2018 Phys. Rev. X 8 031082 |
| [40] | Li H, Chen B B, Chen Z, von Delft J, Weichselbaum A, and Li W 2019 Phys. Rev. B 100 045110 |
| [41] | Carleo G and Troyer M 2017 Science 355 602 |
| [42] | Liao H J, Liu J G, Wang L, and Xiang T 2019 Phys. Rev. X 9 031041 |
| [43] | Chen B B, Gao Y, Guo Y B, Liu Y, Zhao H H, Liao H J, Wang L, Xiang T, Li W, and Xie Z Y 2020 Phys. Rev. B 101 220409(R) |
| [44] | Stoudenmire E and Schwab D J 2016 Advances in Neural Information Processing Systems 29 (Curran Associates, Inc.) p 4799 |
| [45] | Liu D, Ran S J, Wittek P, Peng C, García R B, Su G, and Lewenstein M 2019 New J. Phys. 21 073059 |
| [46] | Ran S J 2019 arXiv:1912.12923 [stat.ML] |
| [47] | Cichocki A, Phan A H, Zhao Q, Lee N, Oseledets I, Sugiyama M, and Mandic D P 2017 Found. Trends$^{\rm\circledR}$ Mach. Learn. 9 431 |
| [48] | Han Z Y, Wang J, Fan H, Wang L, and Zhang P 2018 Phys. Rev. X 8 031012 |
| [49] | Glasser I, Sweke R, Pancotti N, Eisert J, and Cirac J I 2019 33rd Conference on Neural Information Processing Systems, in Advances in Neural Information Processing Systems ed Wallach H, Larochelle H, Beygelzimer A et al. (Vancouver, Canada: Curran Associates, Inc.) vol 32 |
| [50] | Czarnik P and Dziarmaga J 2014 Phys. Rev. B 90 035144 |
| [51] | In Figs. 2 and 3, we first exploit a loss function without the denominator $1/ O^{\exp}_\alpha$, i.e., $\mathcal{L}({\boldsymbol x}) = \sum_{\alpha} \sum_{T > T_{\rm cut}} \lambda_{\alpha} [O^{\exp}_\alpha(T)-O^{{\rm sim},{\boldsymbol x}}_\alpha(T)]^2$, where $\lambda_{\alpha}^{-1/2} = \max_{_{\scriptstyle T>T_{\rm cut}}} [O^{\exp}_\alpha(T), O^{{\rm sim},{\boldsymbol x}}_\alpha(T)]$. Then in Figs. 4 and 5 we follow exactly the loss definition in Eq. (1), and observe that both schemes work well. |
| [52] | LeCun Y, Bengio Y, and Hinton G 2015 Nature 521 436 |
| [53] | Shahriari B, Swersky K, Wang Z, Adams R P, and de Freitas N 2016 Proc. IEEE 104 148 |
| [54] | Melnikov A A, Poulsen N H, Krenn M, Dunjko V, Tiersch M, Zeilinger A, and Briegel H J 2018 Proc. Natl. Acad. Sci. USA 115 1221 |
| [55] | Lizotte D J 2008 PhD Dissertation (Edmonton: University of Alberta) |
| [56] | Bonner J C, Friedberg S A, Kobayashi H, Meier D L, and Blöte H W J 1983 Phys. Rev. B 27 248 |
| [57] | Xu G, Broholm C, Reich D H, and Adams M A 2000 Phys. Rev. Lett. 84 4465 |
| [58] | Xiang J S, Chen C, Li W, Sheng X L, Su N, Cheng Z H, Chen Q, and Chen Z Y 2017 Sci. Rep. 7 44643 |
| [59] | Berger L, Friedberg S A, and Schriempf J T 1963 Phys. Rev. 132 1057 |
| [60] | van Tol M W, Henkens L S J M, and Poulis N J 1971 Phys. Rev. Lett. 27 739 |
| [61] | Li H, Liao Y D, Chen B B, Zeng X T, Sheng X L, Qi Y, Meng Z Y, and Li W 2020 Nat. Commun. 11 1111 |
| [62] | Li Y, Bachus S, Deng H, Schmidt W, Thoma H, Hutanu V, Tokiwa Y, Tsirlin A A, and Gegenwart P 2020 Phys. Rev. X 10 011007 |
| [63] | Shen Y, Liu C, Qin Y, Shen S, Li Y D, Bewley R, Schneidewind A, Chen G, and Zhao J 2019 Nat. Commun. 10 4530 |
| [64] | Cevallos F A, Stolze K, Kong T, and Cava R J 2018 Mater. Res. Bull. 105 154 |
| [65] | Hu Z, Ma Z, Liao Y D, Li H, Ma C, Cui Y, Shangguan Y, Huang Z, Qi Y, Li W, Meng Z Y, Wen J, and Yu W 2020 Nat. Commun. 11 5631 |
| [66] | Dun Z, Daum M, Baral R, Fischer H E, Cao H, Liu Y, Stone M B, Rodriguez-Rivera J A, Choi E S, Huang Q, Zhou H, Mourigal M, and Frandsen B A 2021 Phys. Rev. B 103 064424 |
| [67] | Lou F, Li X Y, Ji J Y, Yu H Y, Feng J S, Gong X G, and Xiang H J 2021 J. Chem. Phys. 154 114103 |
| [68] | Zhitomirsky M E 2003 Phys. Rev. B 67 104421 |
| [69] | Zhitomirsky M E and Honecker A 2004 J. Stat. Mech.: Theory Exp. 2004 P07012 |
| [70] | Garst M and Rosch A 2005 Phys. Rev. B 72 205129 |
| [71] | Wolf B, Tsui Y, Jaiswal-Nagar D, Tutsch U, Honecker A, Remović-Langer K, Hofmann G, Prokofiev A, Assmus W, Donath G, and Lang M 2011 Proc. Natl. Acad. Sci. USA 108 6862 |
| [72] | Gegenwart P 2016 Rep. Prog. Phys. 79 114502 |
| [73] | Karbach P and Stolze J 2005 Phys. Rev. A 72 030301(R) |
| [74] | Cappellaro P, Ramanathan C, and Cory D G 2007 Phys. Rev. Lett. 99 250506 |
|
|
Viewed |
|
|
|
Full text
|
|
|
|
|
Abstract
|
|
|
|
|