| CONDENSED MATTER: ELECTRONIC STRUCTURE, ELECTRICAL, MAGNETIC, AND OPTICAL PROPERTIES |
 |
|

|
|
|
Quantum Spin Liquid Phase in the Shastry–Sutherland Model Detected by an Improved Level Spectroscopic Method |
| Ling Wang1*, Yalei Zhang2, and Anders W. Sandvik3,4* |
1Department of Physics, Zhejiang University, Hangzhou 310000, China
2Beijing Computational Science Research Center, Beijing 100193, China
3Department of Physics, Boston University, Boston, Massachusetts 02215, USA
4Beijing National Laboratory for Condensed Matter Physics and Institute of Physics, Chinese Academy of Sciences, Beijing 100190, China
|
|
| Cite this article: |
|
Ling Wang, Yalei Zhang, and Anders W. Sandvik 2022 Chin. Phys. Lett. 39 077502 |
|
|
|
|
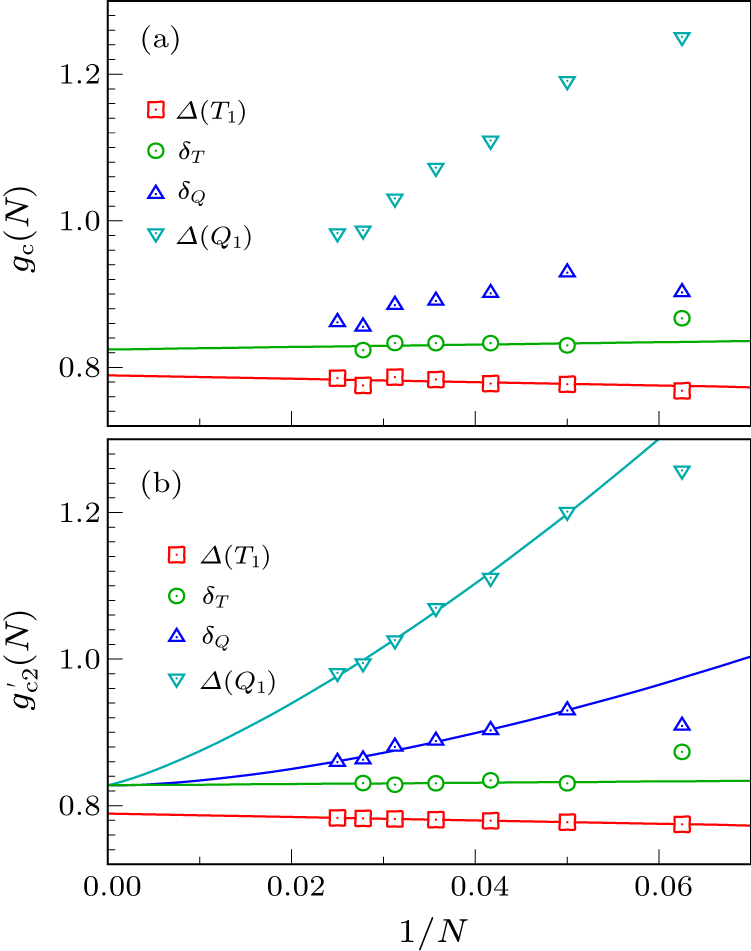
Abstract We study the spin-$1/2$ two-dimensional Shastry–Sutherland spin model by exact diagonalization of clusters with periodic boundary conditions, developing an improved level spectroscopic technique using energy gaps between states with different quantum numbers. The crossing points of some of the relative (composite) gaps have much weaker finite-size drifts than the normally used gaps defined only with respect to the ground state, thus allowing precise determination of quantum critical points even with small clusters. Our results support the picture of a spin liquid phase intervening between the well-known plaquette-singlet and antiferromagnetic ground states, with phase boundaries in almost perfect agreement with a recent density matrix renormalization group study, where much larger cylindrical lattices were used [J. Yang et al., Phys. Rev. B 105, L060409 (2022)]. The method of using composite low-energy gaps to reduce scaling corrections has potentially broad applications in numerical studies of quantum critical phenomena.
|
|
Received: 06 May 2022
Express Letter
Published: 17 June 2022
|
|
| PACS: |
75.10.Jm
|
(Quantized spin models, including quantum spin frustration)
|
| |
75.10.Kt
|
(Quantum spin liquids, valence bond phases and related phenomena)
|
| |
75.40.Mg
|
(Numerical simulation studies)
|
|
|
|
|
|
| [1] | Balents L 2010 Nature 464 199 |
| [2] | Yan S, Huse D A, and White S R 2011 Science 332 1173 |
| [3] | Liao H J, Xie Z Y, Chen J, Liu Z Y, Xie H D, Huang R Z, Normand B, and Xiang T 2017 Phys. Rev. Lett. 118 137202 |
| [4] | He Y C, Zaletel M P, Oshikawa M, and Pollmann F 2017 Phys. Rev. X 7 031020 |
| [5] | Kitaev A 2006 Ann. Phys. 321 2 |
| [6] | Shores M P, Nytko E A, Bartlett B M, and Nocera D G 2005 J. Am. Chem. Soc. 127 13462 |
| [7] | Helton J S et al. 2007 Phys. Rev. Lett. 98 107204 |
| [8] | Chaloupka J, Jackeli G, and Khaliullin G 2010 Phys. Rev. Lett. 105 027204 |
| [9] | Norman M R 2016 Rev. Mod. Phys. 88 041002 |
| [10] | Khuntia P, Velazquez M, Barthélemy Q, Bert F, Kermarrec E, Legros A, Bernu B, Messio L, Zorko A, and Mendels P 2020 Nat. Phys. 16 469 |
| [11] | Zheng J, Ran K, Li T, Wang J, Wang P, Liu B, Liu Z X, Normand B, Wen J, and Yu W 2017 Phys. Rev. Lett. 119 227208 |
| [12] | Li H et al. 2021 Nat. Commun. 12 3513 |
| [13] | Kimchi I, Nahum A, and Senthil T 2018 Phys. Rev. X 8 031028 |
| [14] | Liu L, Shao H, Lin Y C, Guo W, and Sandvik A W 2018 Phys. Rev. X 8 041040 |
| [15] | Kawamura H and Uematsu K 2019 J. Phys.: Condens. Matter 31 504003 |
| [16] | Li Y, Chen G, Tong W, Pi L, Liu J, Yang Z, Wang X, and Zhang Q 2015 Phys. Rev. Lett. 115 167203 |
| [17] | Ma Z et al. 2018 Phys. Rev. Lett. 120 087201 |
| [18] | Kimchi I, Sheckelton J P, McQueen T M, and Lee P A 2018 Nat. Commun. 9 4367 |
| [19] | Kageyama H, Yoshimura K, Stern R, Mushnikov N V, Onizuka K, Kato M, Kosuge K, Slichter C P, Goto T, and Ueda Y 1999 Phys. Rev. Lett. 82 3168 |
| [20] | Waki T, Arai K, Takigawa M, Saiga Y, Uwatoko Y, Kageyama H, and Ueda Y 2007 J. Phys. Soc. Jpn. 76 073710 |
| [21] | Radtke G, Saul A, Dabkowska H A, Salamon M B, and Jaime M 2015 Proc. Natl. Acad. Sci. USA 112 1971 |
| [22] | Haravifard S et al. 2016 Nat. Commun. 7 11956 |
| [23] | Zayed M et al. 2017 Nat. Phys. 13 962 |
| [24] | Bettler S, Stoppel L, Yan Z, Gvasaliya S, and Zheludev A 2020 Phys. Rev. Res. 2 012010(R) |
| [25] | Guo J, Sun G, Zhao B, Wang L, Hong W, Sidorov V A, Ma N, Wu Q, Li S, Meng Z Y, Sandvik A W, and Sun L 2020 Phys. Rev. Lett. 124 206602 |
| [26] | Jiménez J L et al. 2021 Nature 592 370 |
| [27] | Shastry B S and Sutherland B 1981 Physica B+C 108 1069 |
| [28] | Lee J Y, You Y Z, Sachdev S, and Vishwanath A 2019 Phys. Rev. X 9 041037 |
| [29] | Sun G, Ma N, Zhao B, Sandvik A W, and Meng Z Y 2021 Chin. Phys. B 30 067505 |
| [30] | Zhao B, Weinberg P, and Sandvik A W 2019 Nat. Phys. 15 678 |
| [31] | Yang J, Sandvik A W, and Wang L 2022 Phys. Rev. B 105 L060409 |
| [32] | Keleş A and Zhao E 2022 Phys. Rev. B 105 L041115 |
| [33] | Wang L and Sandvik A W 2018 Phys. Rev. Lett. 121 107202 |
| [34] | Gong S S, Zhu W, Sheng D N, Motrunich O I, and Fisher M P A 2014 Phys. Rev. Lett. 113 027201 |
| [35] | Morita S, Kaneko R, and Imada M 2015 J. Phys. Soc. Jpn. 84 024720 |
| [36] | Ferrari F and Becca F 2020 Phys. Rev. B 102 014417 |
| [37] | Nomura Y and Imada M 2021 Phys. Rev. X 11 031034 |
| [38] | Shackleton H, Thomson A, and Sachdev S 2021 Phys. Rev. B 104 045110 |
| [39] | Laflorencie N and Poilblanc D 2004 Quantum Magnetism 645 227 |
| [40] | Noack R M and Manmana S R 2005 AIP Conf. Proc. 789 93 |
| [41] | Weisse A and Fehske H 2008 Computational Many-Particle Physics 739 529 |
| [42] | Läuchli A M 2011 Numerical Simulations of Frustrated Systems, in Introduction to Frustrated Magnetism: Materials, Experiments, Theory. Springer Series in Solid-State Sciences edited by Lacroix C, Mendels P and Mila F (Berlin: Springer) vol 164 pp 481–511 |
| [43] | Sandvik A W 2010 AIP Conf. Proc. 1297 135 |
| [44] | Okamoto K and Nomura K 1992 Phys. Lett. A 169 433 |
| [45] | Eggert S 1996 Phys. Rev. B 54 R9612 |
| [46] | Sandvik A W 2010 Phys. Rev. Lett. 104 137204 |
| [47] | Suwa H, Sen A, and Sandvik A W 2016 Phys. Rev. B 94 144416 |
| [48] | Laflorencie N, Affleck I, and Berciu M 2005 J. Stat. Mech.: Theory Exp. 2005 P12001 |
| [49] | Koga A and Kawakami N 2000 Phys. Rev. Lett. 84 4461 |
| [50] | Corboz P and Mila F 2013 Phys. Rev. B 87 115144 |
| [51] | Anderson P W 1959 Phys. Rev. B 115 2 |
| [52] | Though these triplets with energy between $E(T_{1})$ and $E(T_{2})$ have lattice quantum numbers different from those of $T_{1}$ and $T_{2}$, the corresponding symmetries are not implemented in the DMRG calculation (but the total spin symmetry is implemented in this case), only computed as expectation values with the states obtained. The states have to be generated one-by-one starting from the lowest one and convergence of this procedure becomes increasingly challenging with the number of states computed (Refs.[31, 33]) and we have not been able to reach the state with quantum numbers corresponding to $T_{2}$ for $N=40$. |
| [53] | Nakamura M 2000 Phys. Rev. B 61 16377 |
| [54] | Suwa H and Todo S 2015 Phys. Rev. Lett. 115 080601 |
| [55] | Lecheminant P, Bernu B, Lhuillier C, Pierre L, and Sindzingre P 1997 Phys. Rev. B 56 2521 |
| [56] | Misguich G and Sindzingre P 2007 J. Phys.: Condens. Matter 19 145202 |
| [57] | Schuler M, Whitsitt S, Henry L P, Sachdev S, and Läuchli A M 2016 Phys. Rev. Lett. 117 210401 |
| [58] | Hermele M, Senthil T, and Fisher M P A 2005 Phys. Rev. B 72 104404 |
| [59] | Chen J Y and Poilblanc D 2018 Phys. Rev. B 97 161107(R) |
| [60] | Boyack R, Lin C H, Zerf N, Rayyan A, and Maciejko J 2018 Phys. Rev. B 98 035137 |
| [61] | Dupuis E, Boyack R, and Witczak-Krempa W 2021 arXiv:2108.05922 [cond-mat.str-el] |
| [62] | Liu W Y, Hasik J, Gong S S, Poilblanc D, Chen W Q, and Gu Z C 2021 arXiv:2110.11138 [cond-mat.str-el] |
| [63] | Shackleton H and Sachdev S 2022 arXiv:2203.01962 [cond-mat.str-el] |
| [64] | Xi N, Chen H, Xie Z Y, and Yu R 2021 arXiv:2111.07368 [cond-mat.str-el] |
| [65] | Lu D C, Xu C, and You Y Z 2021 Phys. Rev. B 104 205142 |
| [66] | Cui Y, Liu L, Lin H, Wu K H, Hong W, Liu X, Li C, Hu Z, Xi N, Li S, Yu R, Sandvik A W, and Yu W 2022 arXiv:2204.08133 [cond-mat.str-el] |
|
|
Viewed |
|
|
|
Full text
|
|
|
|
|
Abstract
|
|
|
|
|