| GENERAL |
|

|
|
|
Theory of Critical Phenomena with Memory |
| Shaolong Zeng, Sue Ping Szeto, and Fan Zhong* |
| State Key Laboratory of Optoelectronic Materials and Technologies, School of Physics, Sun Yat-Sen University, Guangzhou 510275, China |
|
| Cite this article: |
|
Shaolong Zeng, Sue Ping Szeto, and Fan Zhong 2022 Chin. Phys. Lett. 39 120501 |
|
|
|
|
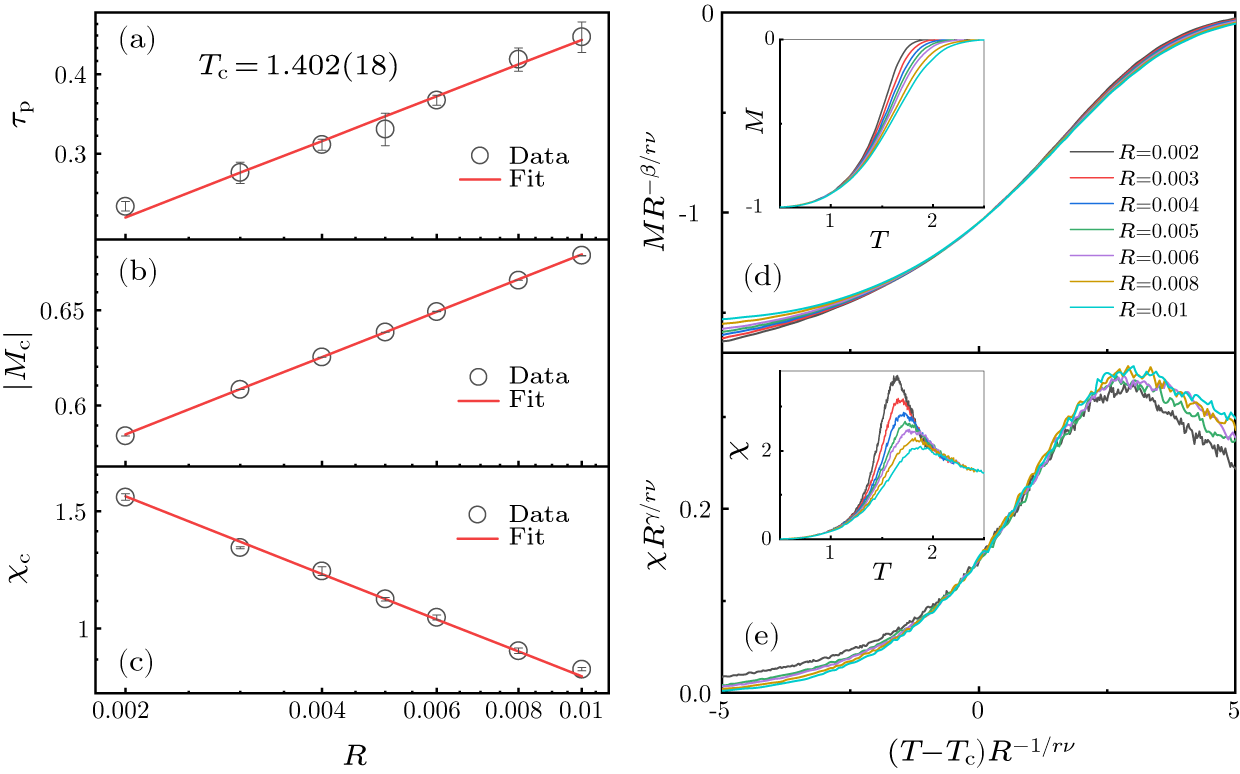
Abstract Memory is a ubiquitous characteristic of complex systems, and critical phenomena are one of the most intriguing phenomena in nature. Here, we propose an Ising model with memory, develop a corresponding theory of critical phenomena with memory for complex systems, and discover a series of surprising novel results. We show that a naive theory of a usual Hamiltonian with a direct inclusion of a power-law decaying long-range temporal interaction violates radically a hyperscaling law for all spatial dimensions even at and below the upper critical dimension. This entails both indispensable consideration of the Hamiltonian for dynamics, rather than the usual practice of just focusing on the corresponding dynamic Lagrangian alone, and transformations that result in a correct theory in which space and time are inextricably interwoven, leading to an effective spatial dimension that repairs the hyperscaling law. The theory gives rise to a set of novel mean-field critical exponents, which are different from the usual Landau ones, as well as new universality classes. These exponents are verified by numerical simulations of the Ising model with memory in two and three spatial dimensions.
|
|
Received: 21 October 2022
Express Letter
Published: 20 November 2022
|
|
| PACS: |
05.50.+q
|
(Lattice theory and statistics)
|
| |
64.60.Ht
|
(Dynamic critical phenomena)
|
| |
64.60.ae
|
(Renormalization-group theory)
|
|
|
|
|
|
| [1] | Ma S K 1976 Modern Theory of Critical Phenomena (Canada: W. A. Benjamin, Inc.) |
| [2] | Cardy J 1996 Scaling and Renormalization in Statistical Physics (Cambridge: Cambridge University Press) |
| [3] | Amit D J and Martin-Mayer V 2005 Field Theory, the Renormalization Group, and Critical Phenomena 3rd edn (Singapore: World Scientific) |
| [4] | Zinn-Justin J 2021 Quantum Field Theory and Critical Phenomena 5th edn (Oxford: Oxford University Press) |
| [5] | Vasil'ev A N 2004 The Field Theoretic Renormalization Group in Critical Behavior Theory and Stochastic Dynamics (London: Chapman and Hall/CRC) |
| [6] | Täuber U C 2014 Critical Dynamics (Cambridge: Cambridge University Press) |
| [7] | Folk R and Moser G 2006 J. Phys. A 39 R207 |
| [8] | Hohenberg P C and Halperin B I 1977 Rev. Mod. Phys. 49 435 |
| [9] | Sachdev S 1999 Quantum Phase Transitions (Cambridge: Cambridge University Press) |
| [10] | Chakravarty S, Ingold G L, Kivelson S, and Luther A 1986 Phys. Rev. Lett. 56 2303 |
| [11] | Werner P, Völker K, Troyer M, and Chakravarty S 2005 Phys. Rev. Lett. 94 047201 |
| [12] | Weiss U 2008 Quantum Dissipative Systems 3rd edn (Singapore: World Scientific) |
| [13] | Yin S, Mai P, and Zhong F 2014 Phys. Rev. B 89 094108 |
| [14] | Caruso F, Giovannetti V, Lupo C, and Mancini S 2014 Rev. Mod. Phys. 86 1203 |
| [15] | Breuer H P, Laine E M, Piilo J, and Vacchini B 2016 Rev. Mod. Phys. 88 021002 |
| [16] | Brown B J, Loss D, Pachos J K, Self C N, and Wootton J R 2016 Rev. Mod. Phys. 88 045005 |
| [17] | Bulla R, Tong N H, and Vojta M 2003 Phys. Rev. Lett. 91 170601 |
| [18] | Winter A, Rieger H, Vojta M, and Bulla R 2009 Phys. Rev. Lett. 102 030601 |
| [19] | Kirchner S, Si Q, and Ingersent K 2009 Phys. Rev. Lett. 102 166405 |
| [20] | Sperstad I B, Stiansen E B, and Sudbø A 2012 Phys. Rev. B 85 214302 |
| [21] | De Filippis G, de Candia A, Cangemi L M, Sassetti M, Fazio R, and Cataudella V 2020 Phys. Rev. B 101 180408(R) |
| [22] | Wang Y Z, He S, Duan L, and Chen Q H 2021 Phys. Rev. B 103 205106 |
| [23] | Schulz M, Trimper S, and Zabrocki K 2007 J. Phys. A 40 3369 |
| [24] | Tarasov V E and Zaslavsky G M 2007 Phys. A 383 291 |
| [25] | Bouchaud J P and Georges A 1990 Phys. Rep. 195 127 |
| [26] | Metzler R and Klafter J 2000 Phys. Rep. 339 1 |
| [27] | West B J 2014 Rev. Mod. Phys. 86 1169 |
| [28] | Keim N C, Paulsen J D, Zeravcic Z, Sastry S, and Nagel S R 2019 Rev. Mod. Phys. 91 035002 |
| [29] | Tsimring L S and Pikovsky A 2001 Phys. Rev. Lett. 87 250602 |
| [30] | Masoller C 2002 Phys. Rev. Lett. 88 034102 |
| [31] | Masoller C 2003 Phys. Rev. Lett. 90 020601 |
| [32] | Trimper S, Zabrocki K, and Schulz M 2002 Phys. Rev. E 66 026114 |
| [33] | Schütz G M and Trimper S 2004 Phys. Rev. E 70 045101(R) |
| [34] | Trimper S, Zabrocki K, and Schulz M 2004 Phys. Rev. E 70 056133 |
| [35] | Mokshin A V, Yulmetyev R M, and Hänggi P 2005 Phys. Rev. Lett. 95 200601 |
| [36] | Scalliet C and Berthier L 2019 Phys. Rev. Lett. 122 255502 |
| [37] | Jack R L and Harris R J 2020 Phys. Rev. E 102 012154 |
| [38] | Narinder N, Bechinger C, and Gomez-Solano J R 2018 Phys. Rev. Lett. 121 078003 |
| [39] | Lozano C, Gomez-Solano J R, and Bechinger C 2019 Nat. Mater. 18 1118 |
| [40] | Pastor-Satorras R, Castellano C, Van Mieghem P, and Vespignani A 2015 Rev. Mod. Phys. 87 925 |
| [41] | Van Mieghem P and van de Bovenkamp R 2013 Phys. Rev. Lett. 110 108701 |
| [42] | Gleeson J P, O'Sullivan K P, Baños R A, and Moreno Y 2016 Phys. Rev. X 6 021019 |
| [43] | Lin Z H, Feng M, Tang M, Liu Z, Xu C, Hui P M, and Lai Y C 2020 Nat. Commun. 11 2490 |
| [44] | Zaslavsky G M 2005 Hamiltonian Chaos and Fractional Dynamics (Oxford: Oxford University Press) |
| [45] | Rangarajan G and Ding M (eds) 2003 Processes with Long-Range Correlations: Theory and Applications, Lecture Notes in Physics vol 621 (Berlin: Springer-Verlag) |
| [46] | Beran J, Feng Y, Ghosh S, and Kulik R 2013 Long-Memory Processes: Probabilistic Properties and Statistical Methods (Berlin: Springer-Verlag) |
| [47] | Wunsch C 2015 Modern Observational Physical Oceanography: Understanding the Global Ocean (Princeton, NJ: Princeton University Press) |
| [48] | Valagiannopoulos C, Sarsen A, and Alù A 2021 IEEE Trans. Anten. Propag. 69 7720 |
| [49] | Valagiannopoulos C 2022 IEEE Trans. Anten. Propag. 70 5534 |
| [50] | Murray J D 2000 Mathematical Biology, part I (Berlin: Springer) |
| [51] | Murray J D 2003 Mathematical Biology, part II (Berlin: Springer) |
| [52] | Kappler J, Daldrop J O, Brünig F N, Boehle M D, and Netz R R 2018 J. Chem. Phys. 148 014903 |
| [53] | Freeman M 2000 Nature 408 313 |
| [54] | Zhang J J and Zhou T 2019 Proc. Natl. Acad. Sci. USA 116 23542 |
| [55] | Jiang Z J, Zhou J W, Hou T Q, Wong K Y M, and Huang H P 2021 Phys. Rev. E 104 064306 |
| [56] | Campa A, Dauxois T, and Ruffo S 2009 Phys. Rep. 480 57 |
| [57] | Fisher M E, Ma S K, and Nickel B G 1972 Phys. Rev. Lett. 29 917 |
| [58] | Jurcevic P, Lanyon B P, Hauke P, Hempel C, Zoller P, Blatt R, and Roos C F 2014 Nature 511 202 |
| [59] | Britton J W, Sawyer B C, Keith A C, Wang C C J, Freericks J K, Uys H, Biercuk M J, and Bollinger J J 2012 Nature 484 489 |
| [60] | Islam R, Senko C, Campbell W, Korenblit S, Smith J, Lee A, Edwards E, Wang C C, Freericks J, and Monroe C 2013 Science 340 583 |
| [61] | Richerme P, Gong Z X, Lee A, Senko C, Smith J, Foss-Feig M, Michalakis S, Gorshkov A V, and Monroe C 2014 Nature 511 198 |
| [62] | Bohnet J G, Sawyer B C, Britton J W, Wall M L, Rey A M, Foss-Feig M, and Bollinger J J 2016 Science 352 1297 |
| [63] | Yang F, Jiang S J, and Zhou F 2019 Phys. Rev. A 99 012119 |
| [64] | Metropolis N, Rosenbluth A W, Rosenbluth M N, Teller A M, and Teller E 1953 J. Chem. Phys. 21 1087 |
| [65] | Landau D P and Binder K 2005 A Guide to Monte Carlo Simulations in Statistical Physics 2nd edn (Cambridge: Cambridge University Press) |
| [66] | Glauber R J 1963 J. Math. Phys. 4 294 |
| [67] | Gong S R, Zhong F, Huang X Z, and Fan S L 2010 New J. Phys. 12 043036 |
| [68] | Zhong F 2011 Applications of Monte Carlo Method in Science and Engineering, edited by Mordechai S (Intech, Rijeka, Croatia) p 469 available at http://www.dwz.cn/B9Pe2 |
| [69] | Feng B Q, Yin S, and Zhong F 2016 Phys. Rev. B 94 144103 |
| [70] | Zeng S S, Szeto S P, and Zhong F 2022 arXiv:2203.16243 [cond-mat.stat-mech] for a proof of the equivalence of the two models |
| [71] | Janssen H K 1979 Dynamical Critical Phenomena and Related topics, Enz C P (ed) Lecture Notes in Physics vol 104 (Berlin: Springer) |
| [72] | Janssen H K 1992 From Phase Transition to Chaos, Györgyi G, Kondor I, Sasvári L, and Tél T (eds) (Singapore: World Scientific) |
| [73] | Martin P C, Siggia E D, and Rose H A 1973 Phys. Rev. A 8 423 |
| [74] | Sak J 1973 Phys. Rev. B 8 281 |
| [75] | It is derived from the standard scaling laws $d\nu=2-\alpha$, $\alpha+2\beta+\gamma=2$, and $\gamma=\beta(\delta-1)$ (see Refs. [1-5]) |
| [76] | Zhong F 2017 Front. Phys. 12 126402 |
| [77] | In the long-range fixed point $v^{*}\neq0$ and to the present one-loop order, Eq. () is equal to $\gamma_{\lambda_{1}}|=2-2/\theta$ rather than zero because it is irrlevant (see the text). However, to higher orders, it is again equal to zero and leads to crossover, see Ref. [78] for details. |
| [78] | Zeng S, Szeto S P, and Zhong F 2022 in preparation |
| [79] | Note that although $\gamma_{t}$ becomes $\gamma_{t'}=\gamma_{t}-\gamma_{a}/2$, $d$ also changes to $d-\gamma_{a}/2$. Consequently, $\gamma_{h}$ keeps unchanged. |
| [80] | Yin S, Qin X, Lee C, and Zhong F 2012 arXiv:1207.1602 [cond-mat.stat-mech] |
| [81] | Liu C W, Polkovnikov A, and Sandvik A W 2014 Phys. Rev. B 89 054307 |
| [82] | Huang Y Y, Yin S, Feng B Q, and Zhong F 2014 Phys. Rev. B 90 134108 |
| [83] | Liu C W, Polkovnikov A, Sandvik A W, and Young A P 2015 Phys. Rev. E 92 022128 |
| [84] | Liu C W, Polkovnikov A, and Sandvik A W 2015 Phys. Rev. Lett. 114 147203 |
| [85] | Huang Y Y, Yin S, Hu Q J, and Zhong F 2016 Phys. Rev. B 93 024103 |
| [86] | Pelissetto A and Vicari E 2016 Phys. Rev. E 93 032141 |
| [87] | Xu N, Castelnovo C, Melko R G, Chamon C, and Sandvik A W 2018 Phys. Rev. B 97 024432 |
| [88] | Xue M, Yin S, and You L 2018 Phys. Rev. A 98 013619 |
| [89] | Cao X M, Hu Q J, and Zhong F 2018 Phys. Rev. B 98 245124 |
| [90] | Gerster M, Haggenmiller B, Tschirsich F, Silvi P, and Montangero S 2019 Phys. Rev. B 100 024311 |
| [91] | Li Y H, Zeng Z D, and Zhong F 2019 Phys. Rev. E 100 020105(R) |
| [92] | Mathey S and Diehl S 2020 Phys. Rev. Res. 2 013150 |
| [93] | Yuan W L, Yin S, and Zhong F 2021 Chin. Phys. Lett. 38 026401 |
| [94] | Yuan W L and Zhong F 2021 J. Phys.: Condens. Matter 33 375401 |
| [95] | Yuan W L and Zhong F 2021 J. Phys.: Condens. Matter 33 385401 |
| [96] | Zuo Z Y, Yin S, Cao X M, and Zhong F 2021 Phys. Rev. B 104 214108 |
| [97] | Clark L W, Feng L, and Chin C 2016 Science 354 606 |
| [98] | Keesling A, Omran A, Levine H, Bernien H, Pichler H, Choi S, Samajdar R, Schwartz S, Silvi P, Sachdev S, Zoller P, Endres M, Greiner M, Vuletić V, and Lukin M D 2019 Nature 568 207 |
| [99] | Zhong F 2002 Phys. Rev. B 66 060401(R) |
| [100] | Zhong F 2006 Phys. Rev. E 73 047102 |
| [101] | Luijten E and Blöte H W J 1997 Phys. Rev. B 56 8945 |
|
|
Viewed |
|
|
|
Full text
|
|
|
|
|
Abstract
|
|
|
|
|