| CONDENSED MATTER: ELECTRONIC STRUCTURE, ELECTRICAL, MAGNETIC, AND OPTICAL PROPERTIES |
 |
|

|
|
|
Variational Corner Transfer Matrix Renormalization Group Method for Classical Statistical Models |
| X. F. Liu1†, Y. F. Fu1†, W. Q. Yu1, J. F. Yu2*, and Z. Y. Xie1* |
1Department of Physics, Renmin University of China, Beijing 100872, China
2School of Physics and Electronics, Hunan University, Changsha 410082, China
|
|
| Cite this article: |
|
X. F. Liu, Y. F. Fu, W. Q. Yu et al 2022 Chin. Phys. Lett. 39 067502 |
|
|
|
|
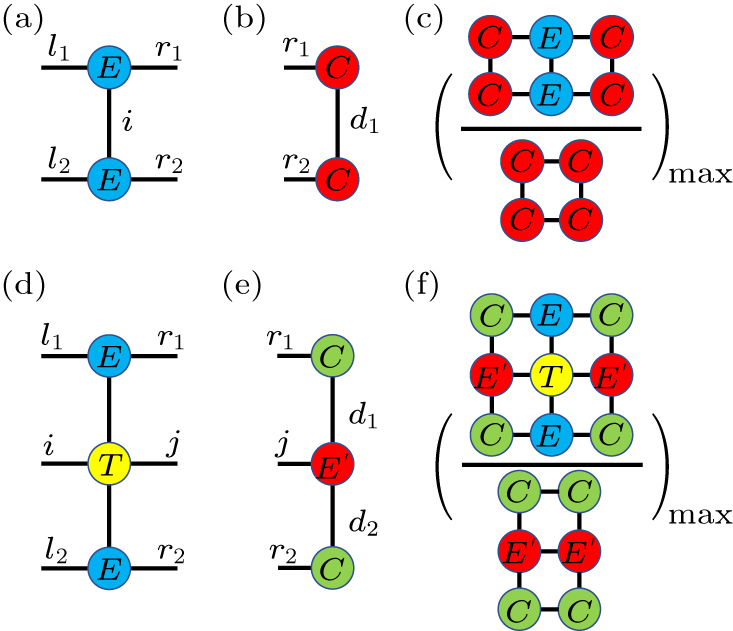
Abstract In the context of tensor network states, we for the first time reformulate the corner transfer matrix renormalization group (CTMRG) method into a variational bilevel optimization algorithm. The solution of the optimization problem corresponds to the fixed-point environment pursued in the conventional CTMRG method, from which the partition function of a classical statistical model, represented by an infinite tensor network, can be efficiently evaluated. The validity of this variational idea is demonstrated by the high-precision calculation of the residual entropy of the dimer model, and is further verified by investigating several typical phase transitions in classical spin models, where the obtained critical points and critical exponents all agree with the best known results in literature. Its extension to three-dimensional tensor networks or quantum lattice models is straightforward, as also discussed briefly.
|
|
Received: 31 March 2022
Express Letter
Published: 11 May 2022
|
|
| PACS: |
05.10.Cc
|
(Renormalization group methods)
|
|
|
|
|
|
| [1] | Kosterlitz J M and Thouless D J 1973 J. Phys. C 6 1181 |
| [2] | Kosterlitz J M 1974 J. Phys. C 7 1046 |
| [3] | Metropolis N and Ulam S 1949 J. Am. Stat. Assoc. 44 335 |
| [4] | White S R 1992 Phys. Rev. Lett. 69 2863 |
| [5] | White S R 1993 Phys. Rev. B 48 10345 |
| [6] | Verstraete F and Cirac J I 2004 arXiv:cond-mat/0407066 [cond-mat.str-el] |
| [7] | Zhao H H, Xie Z Y, Chen Q N, Wei Z C, Cai J W, and Xiang T 2010 Phys. Rev. B 81 174411 |
| [8] | Orús R 2019 Nat. Rev. Phys. 1 538 |
| [9] | Liao H J, Xie Z Y, Chen J, Liu Z Y, Xie H D, Huang R Z, Normand B, and Xiang T 2017 Phys. Rev. Lett. 118 137202 |
| [10] | Mei J W, Chen J Y, He H, and Wen X G 2017 Phys. Rev. B 95 235107 |
| [11] | Wang L, Gu Z C, Verstraete F, and Wen X G 2016 Phys. Rev. B 94 075143 |
| [12] | LeBlanc J P F, Antipov A E, Becca F, Bulik I W, Chan G K L, Chung C M, Deng Y, Ferrero M, Henderson T M, Jiménez-Hoyos C A, Kozik E, Liu X W, Millis A J, Prokof'ev N V, Qin M, Scuseria G E, Shi H, Svistunov B V, Tocchio L F, Tupitsyn I S, White S R, Zhang S, Zheng B X, Zhu Z, and Gull E (Simons Collaboration on the Many-Electron Problem) 2015 Phys. Rev. X 5 041041 |
| [13] | Corboz P, Rice T M, and Troyer M 2014 Phys. Rev. Lett. 113 046402 |
| [14] | Xie Z Y, Chen J, Qin M P, Zhu J W, Yang L P, and Xiang T 2012 Phys. Rev. B 86 045139 |
| [15] | Yu J F, Xie Z Y, Meurice Y, Liu Y, Denbleyker A, Zou H, Qin M P, Chen J, and Xiang T 2014 Phys. Rev. E 89 013308 |
| [16] | Wang C, Qin S M, and Zhou H J 2014 Phys. Rev. B 90 174201 |
| [17] | Ran S J, Tirrito E, Peng C, Chen X, Tagliacozzo L, Su G, and Lewenstein M 2020 Tensor Network Contractions: Methods and Applications to Quantum Many-Body Systems (Springer Nature) |
| [18] | Levin M and Nave C P 2007 Phys. Rev. Lett. 99 120601 |
| [19] | Xie Z Y, Jiang H C, Chen Q N, Weng Z Y, and Xiang T 2009 Phys. Rev. Lett. 103 160601 |
| [20] | Gu Z C and Wen X G 2009 Phys. Rev. B 80 155131 |
| [21] | Evenbly G and Vidal G 2015 Phys. Rev. Lett. 115 180405 |
| [22] | Yang S, Gu Z C, and Wen X G 2017 Phys. Rev. Lett. 118 110504 |
| [23] | Bal M, Mariën M, Haegeman J, and Verstraete F 2017 Phys. Rev. Lett. 118 250602 |
| [24] | Vidal G 2003 Phys. Rev. Lett. 91 147902 |
| [25] | Orús R and Vidal G 2008 Phys. Rev. B 78 155117 |
| [26] | Zauner-Stauber V, Vanderstraeten L, Fishman M T, Verstraete F, and Haegeman J 2018 Phys. Rev. B 97 045145 |
| [27] | Nishino T and Okunishi K 1996 J. Phys. Soc. Jpn. 65 891 |
| [28] | Orús R and Vidal G 2009 Phys. Rev. B 80 094403 |
| [29] | Fishman M T, Vanderstraeten L, Zauner-Stauber V, Haegeman J, and Verstraete F 2018 Phys. Rev. B 98 235148 |
| [30] | Baxter R J 1982 Exactly Solved Models in Statistical Mechanics (London: Academic Press) |
| [31] | Corboz P, Lajkó M, Läuchli A M, Penc K, and Mila F 2012 Phys. Rev. X 2 041013 |
| [32] | Xie Z Y, Liao H J, Huang R Z, Xie H D, Chen J, Liu Z Y, and Xiang T 2017 Phys. Rev. B 96 045128 |
| [33] | Jahromi S S, Orús R, Kargarian M, and Langari A 2018 Phys. Rev. B 97 115161 |
| [34] | Kasteleyn P W 1963 J. Math. Phys. 4 287 |
| [35] | Vanderstraeten L, Vanhecke B, and Verstraete F 2018 Phys. Rev. E 98 042145 |
| [36] | Potts R B 1952 Math. Proc. Cambridge Philos. Soc. 48 106 |
| [37] | Wu F Y 1982 Rev. Mod. Phys. 54 235 |
| [38] | José J V, Kadanoff L P, Kirkpatrick S, and Nelson D R 1977 Phys. Rev. B 16 1217 |
| [39] | Tomita Y and Okabe Y 2002 Phys. Rev. B 65 184405 |
| [40] | Rastelli E, Regina S, and Tassi A 2004 Phys. Rev. B 69 174407 |
| [41] | Borisenko O, Cortese G, Fiore R, Gravina M, and Papa A 2011 Phys. Rev. E 83 041120 |
| [42] | Kramers H A and Wannier G H 1941 Phys. Rev. 60 252 |
| [43] | Chen J, Liao H J, Xie H D, Han X J, Huang R Z, Cheng S, Wei Z C, Xie Z Y, and Xiang T 2017 Chin. Phys. Lett. 34 050503 |
| [44] | Suzuki M 1967 Prog. Theor. Phys. 37 770 |
| [45] | Chatelain C 2014 J. Stat. Mech.: Theory Exp. 2014 P11022 |
| [46] | Chen Y, Xie Z Y, and Yu J F 2018 Chin. Phys. B 27 080503 |
| [47] | Li Z Q, Yang L P, Xie Z Y, Tu H H, Liao H J, and Xiang T 2020 Phys. Rev. E 101 060105 |
| [48] | Chen Y, Ji K, Xie Z Y, and Yu J F 2020 Phys. Rev. B 101 165123 |
| [49] | Nishino T and Okunishi K 1998 J. Phys. Soc. Jpn. 67 3066 |
| [50] | Orús R 2012 Phys. Rev. B 85 205117 |
|
|
Viewed |
|
|
|
Full text
|
|
|
|
|
Abstract
|
|
|
|
|